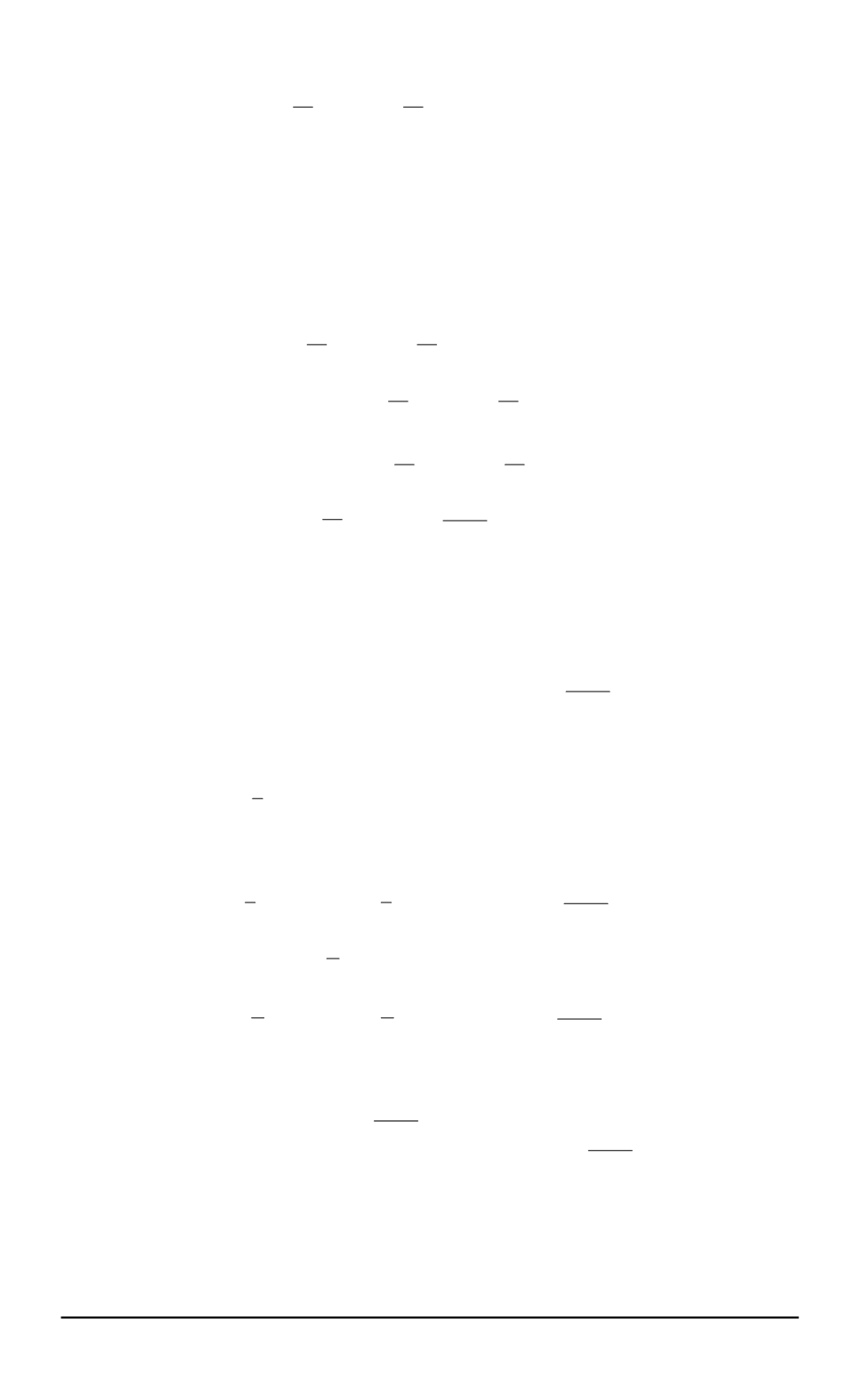
2)
the solution
η
(
t
)
of the Cauchy problem
˙
η
=
q
(
B
1
(
t
)
, . . . , B
m
(
t
)
, η
)
, η
(0) =
η
0
(5)
is determined at all
t
∈
[0
, t
∗
]
and satisfies the condition
η
(
t
∗
) =
η
∗
.
(6)
In paper [9] it is also shown that the control
u
=
u
(
t
)
, which is the
solution to the terminal problem, is found according to the equality
u
(
t
) =
g
−
1
(
B
1
(
t
)
, . . . , B
m
(
t
)
, η
(
t
))
×
×
B
(
r
1
)
1
(
t
)
−
f
1
(
B
1
(
t
)
, . . . , B
m
(
t
)
, η
(
t
))
. . .
B
(
r
m
)
m
(
t
)
−
f
m
(
B
1
(
t
)
, . . . , B
m
(
t
)
, η
(
t
))
,
(7)
while the relations
z
i
=
B
i
(
t
)
,
i
= 1
, m
,
η
=
η
(
t
)
,
t
∈
[0
, t
∗
]
are the
parametric equations of that phase trajectory of system (2) which connects
states (3) and (4).
According to [9], we shall find functions
B
1
(
t
)
, . . . , B
m
(
t
)
from
theorem 2 in the form of
B
i
(
t
) =
b
i
(
t
) +
c
i
d
i
(
t
)
,
i
= 1
, m,
where
b
i
(
t
)
,
d
i
(
t
)
∈
C
r
i
([0
, t
∗
])
, the vector-functions
b
i
(
t
) =
b
i
(
t
)
, b
0
i
(
t
)
, . . . , b
(
r
i
−
1)
i
(
t
)
T
satisfy the conditions
b
i
(0) =
z
i
0
, b
i
(
t
∗
) =
z
i
∗
, i
= 1
, m,
while the vector-functions
d
i
(
t
) =
d
i
(
t
)
, d
0
i
(
t
)
, . . . , d
(
r
i
−
1)
i
(
t
)
T
satisfy the
conditions
d
i
(0) = 0
, d
i
(
t
∗
) = 0
, i
= 1
, m,
(8)
It is necessary to find
c
i
∈
R
.
It is possible, for example, to take interpolation polynomials of
2
r
i
−
1
degrees as functions
b
i
(
t
)
,
i
= 1
, m
, and to take any functions, for which
correlations (8) are fulfilled as functions
d
i
(
t
)
,
i
= 1
, m
. With the given
set of functions
B
i
(
t
)
, condition 1 of theorem 2 is fulfilled for any
c
i
∈
R
.
Numbers
c
i
should be selected in such a way that condition 2 of theorem 2
was fulfilled. If there exist such numbers as
c
1
=
c
1
∗
, . . . , c
m
=
c
m
∗
that the
solution
η
(
t
)
of the Cauchy problem (5) satisfies the additional requirement
ISSN 1812-3368. Herald of the BMSTU. Series “Natural Sciences”. 2014. No. 5
19